How Do You Know When a Parabola Is Negative
Learning Outcomes
- Identify the vertex, centrality of symmetry, [latex]y[/latex]-intercept, and minimum or maximum value of a parabola from it's graph.
- Identify a quadratic function written in general and vertex form.
- Given a quadratic function in general form, find the vertex.
- Define the domain and range of a quadratic role by identifying the vertex every bit a maximum or minimum.
The graph of a quadratic role is a U-shaped curve chosen a parabola. One important characteristic of the graph is that information technology has an farthermost point, called the vertex. If the parabola opens up, the vertex represents the lowest point on the graph, or the minimum value of the quadratic function. If the parabola opens down, the vertex represents the highest point on the graph, or the maximum value. In either example, the vertex is a turning point on the graph. The graph is likewise symmetric with a vertical line drawn through the vertex, called the axis of symmetry.

The [latex]y[/latex]-intercept is the bespeak at which the parabola crosses the [latex]y[/latex]-axis. The [latex]x[/latex]-intercepts are the points at which the parabola crosses the [latex]10[/latex]-axis. If they be, the [latex]ten[/latex]-intercepts represent the zeros, or roots, of the quadratic role, the values of [latex]x[/latex] at which [latex]y=0[/latex].
Example: Identifying the Characteristics of a Parabola
Determine the vertex, axis of symmetry, zeros, and y-intercept of the parabola shown below.
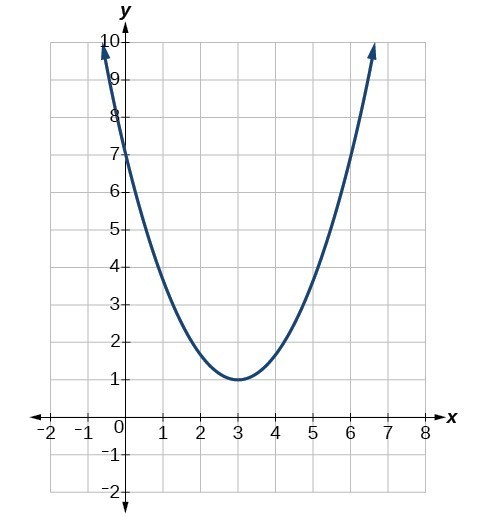
Testify Solution
The vertex is the turning point of the graph. We can see that the vertex is at [latex](3,i)[/latex]. The axis of symmetry is the vertical line that intersects the parabola at the vertex. So the axis of symmetry is [latex]x=3[/latex]. This parabola does not cross the [latex]x[/latex]-axis, so it has no zeros. Information technology crosses the [latex]y[/latex]-centrality at (0, 7) so this is the [latex]y[/latex]-intercept.
Equations of Quadratic Functions
The general course of a quadratic function presents the function in the course
[latex]f\left(10\right)=a{x}^{2}+bx+c[/latex]
where [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex] are real numbers and [latex]a\ne 0[/latex]. If [latex]a>0[/latex], the parabola opens upward. If [latex]a<0[/latex], the parabola opens downward. We can employ the general form of a parabola to observe the equation for the axis of symmetry.
The axis of symmetry is defined by [latex]x=-\dfrac{b}{2a}[/latex]. If nosotros apply the quadratic formula, [latex]x=\dfrac{-b\pm \sqrt{{b}^{two}-4ac}}{2a}[/latex], to solve [latex]a{ten}^{2}+bx+c=0[/latex] for the [latex]x[/latex]-intercepts, or zeros, we find the value of [latex]x[/latex] halfway between them is ever [latex]x=-\dfrac{b}{2a}[/latex], the equation for the axis of symmetry.
The figure beneath shows the graph of the quadratic function written in general class as [latex]y={ten}^{2}+4x+3[/latex]. In this form, [latex]a=ane,\text{ }b=4[/latex], and [latex]c=3[/latex]. Because [latex]a>0[/latex], the parabola opens upward. The axis of symmetry is [latex]x=-\dfrac{4}{two\left(1\right)}=-ii[/latex]. This too makes sense considering we can see from the graph that the vertical line [latex]x=-2[/latex] divides the graph in one-half. The vertex always occurs forth the axis of symmetry. For a parabola that opens upwardly, the vertex occurs at the everyman point on the graph, in this instance, [latex]\left(-ii,-1\correct)[/latex]. The [latex]x[/latex]-intercepts, those points where the parabola crosses the [latex]x[/latex]-axis, occur at [latex]\left(-iii,0\right)[/latex] and [latex]\left(-1,0\right)[/latex].

The standard form of a quadratic function presents the part in the form
[latex]f\left(x\right)=a{\left(x-h\right)}^{2}+1000[/latex]
where [latex]\left(h,\text{ }one thousand\right)[/latex] is the vertex. Because the vertex appears in the standard form of the quadratic part, this form is also known equally the vertex course of a quadratic function.
Given a quadratic function in general grade, find the vertex of the parabola.
I reason we may want to identify the vertex of the parabola is that this point will inform us where the maximum or minimum value of the output occurs, [latex]thousand[/latex], and where it occurs, [latex]h[/latex]. If we are given the general form of a quadratic role:
[latex]f(x)=ax^2+bx+c[/latex]
We tin can define the vertex, [latex](h,g)[/latex], by doing the post-obit:
- Identify [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex].
- Find [latex]h[/latex], the [latex]x[/latex]-coordinate of the vertex, past substituting [latex]a[/latex] and [latex]b[/latex] into [latex]h=-\dfrac{b}{2a}[/latex].
- Find [latex]k[/latex], the [latex]y[/latex]-coordinate of the vertex, by evaluating [latex]grand=f\left(h\correct)=f\left(-\dfrac{b}{2a}\correct)[/latex]
Case: Finding the Vertex of a Quadratic Function
Find the vertex of the quadratic function [latex]f\left(ten\right)=2{x}^{2}-6x+7[/latex]. Rewrite the quadratic in standard course (vertex form).
Endeavour It
Given the equation [latex]g\left(x\correct)=13+{ten}^{ii}-6x[/latex], write the equation in general form and then in standard form.
Show Solution
[latex]g\left(x\correct)={x}^{2}-6x+thirteen[/latex] in full general form; [latex]one thousand\left(x\right)={\left(x - three\right)}^{2}+4[/latex] in standard grade
Finding the Domain and Range of a Quadratic Role
Any number tin be the input value of a quadratic function. Therefore the domain of any quadratic function is all real numbers. Because parabolas take a maximum or a minimum at the vertex, the range is restricted. Since the vertex of a parabola volition be either a maximum or a minimum, the range volition consist of all [latex]y[/latex]-values greater than or equal to the [latex]y[/latex]-coordinate of the vertex or less than or equal to the [latex]y[/latex]-coordinate at the turning betoken, depending on whether the parabola opens upwardly or down.
A General Note: Domain and Range of a Quadratic Function
The domain of whatsoever quadratic function is all real numbers.
The range of a quadratic part written in general form [latex]f\left(x\right)=a{ten}^{ii}+bx+c[/latex] with a positive [latex]a[/latex] value is [latex]f\left(x\right)\ge f\left(-\frac{b}{2a}\correct)[/latex], or [latex]\left[f\left(-\frac{b}{2a}\correct),\infty \right)[/latex]; the range of a quadratic office written in general form with a negative [latex]a[/latex] value is [latex]f\left(x\right)\le f\left(-\frac{b}{2a}\correct)[/latex], or [latex]\left(-\infty ,f\left(-\frac{b}{2a}\right)\correct][/latex].
The range of a quadratic role written in standard form [latex]f\left(x\right)=a{\left(x-h\correct)}^{2}+k[/latex] with a positive [latex]a[/latex] value is [latex]f\left(10\correct)\ge k[/latex]; the range of a quadratic function written in standard grade with a negative [latex]a[/latex] value is [latex]f\left(x\correct)\le thousand[/latex].
How To: Given a quadratic function, find the domain and range.
- The domain of whatever quadratic office as all real numbers.
- Determine whether [latex]a[/latex] is positive or negative. If [latex]a[/latex] is positive, the parabola has a minimum. If [latex]a[/latex] is negative, the parabola has a maximum.
- Decide the maximum or minimum value of the parabola, [latex]k[/latex].
- If the parabola has a minimum, the range is given by [latex]f\left(x\right)\ge k[/latex], or [latex]\left[k,\infty \right)[/latex]. If the parabola has a maximum, the range is given by [latex]f\left(x\right)\le chiliad[/latex], or [latex]\left(-\infty ,k\correct][/latex].
Instance: Finding the Domain and Range of a Quadratic Function
Find the domain and range of [latex]f\left(x\correct)=-5{ten}^{2}+9x - one[/latex].
Attempt It
Find the domain and range of [latex]f\left(ten\correct)=2{\left(ten-\dfrac{4}{vii}\right)}^{2}+\dfrac{8}{11}[/latex].
Testify Solution
The domain is all real numbers. The range is [latex]f\left(x\right)\ge \dfrac{8}{11}[/latex], or [latex]\left[\dfrac{8}{11},\infty \right)[/latex].
Contribute!
Did yous accept an idea for improving this content? We'd dear your input.
Ameliorate this pageLearn More
Source: https://courses.lumenlearning.com/waymakercollegealgebra/chapter/characteristics-of-parabolas/

0 Response to "How Do You Know When a Parabola Is Negative"
Post a Comment